达朗贝尔原理

D'Alembertsche Trägheitskraft an einem bewegten Körper

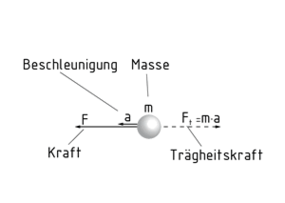
达朗贝尔原理是指采用静态的方法来计算动态系统的问题。由于物体的合外力${{F}_{R}}$与惯性力${{F}_{\tau }}$相对应，所以引用虚构的惯性力 ${{F}_{\tau }}$ （达朗贝尔辅助力）可以建立系统的平衡。

由动态系统的质量m引起的力称为惯性力，它作用在系统的重心位置。惯性力的作用方向与物体加速度a和运动方向相反，它可由质量m与加速度值a计算得到。假设目标系统沿正方向行进，那么在计算惯性力的等式前应添加负号。应用如下： ${{F}_{\tau }}=-m\cdot a$

运动物体的达朗贝尔辅助力

D'Alembertsches Prinzip

D'Alembertsche Trägheitskraft an einem bewegten Körper

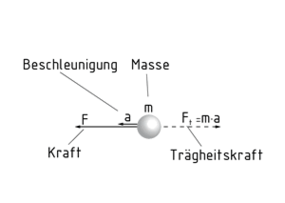
Das Prinzip von d'Alembert ermöglicht die Berechnung eines dynamischen Systems unter statischer Betrachtungsweise. Die Einführung der d'Alembertschen Trägheitskraft ${{F}_{\tau }}$ als eine sogenannte Scheinkraft bringt das System in ein Gleichgewicht, da die Summe der äußeren Kräfte ${{F}_{R}}$ betragsmäßig der Trägheitskraft ${{F}_{\tau }}$ entspricht.

Die Trägheitskraft wird durch die Masse m des bewegten Systems verursacht und greift deshalb in dessen Schwerpunkt an. Entgegengesetzt der Beschleunigung a und somit entgegengesetzt der Richtung der Bewegung des Systems, ist die Trägheitskraft gerichtet. Sie ist das Produkt aus Masse m und Beschleunigung a. Da die Bewegungsrichtung des zu berechnenden Systems als positiv angenommen wird, hat die Trägheitskraft ein negatives Vorzeichen. Es gilt: ${{F}_{\tau }}=-m\cdot a$

D'Alembert's principle

D'Alembert's auxiliary force on a body in motion

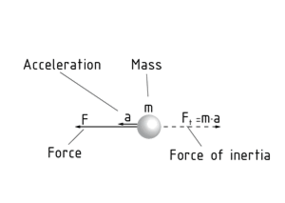
The principle of d'Alembert is used to calculate a dynamic system from a static perspective. Introducing a Force of inertia ${{F}_{\tau }}$ (d'Alembert's auxiliary force) as a fictitious force creates an Equilibrium in the system, because the sum of the external forces ${{F}_{R}}$ corresponds to the force of inertia ${{F}_{\tau }}$.

The force of inertia is caused by the mass m of the dynamic system and therefore acts at its Centre of gravity. The force of inertia acts in the opposite direction to the acceleration a and thus to the system's motion. It is the product of mass m and acceleration a. Since the system to be calculated is assumed to be moving in a positive direction, the force of inertia is preceded by a negative sign. The following applies: ${{F}_{\tau }}=-m\cdot a$

Principio de D'Alembert

Fuerza auxiliar de d'Alembert en un cuerpo en movimiento

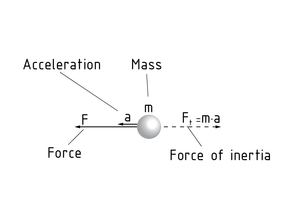
El principio de D'Alembert se usa para calcular un sistema dinámico desde un punto de vista estático. El introducir una fuerza de inercia ${{F}_{\tau }}$ (fuerza auxiliar de D?Alembert) como una fuerza ficticia, se crea un equilibrio en el sistema, ya que la suma de las fuerzas externas ${{F}_{R}}$ corresponde a la fuerza de inercia ${{F}_{\tau }}$ .

La fuerza de inercia es causada por la masa m del sistema dinámico y por lo tanto actúa en su centro de gravedad. La fuerza de inercia actúa en la dirección opuesta a la aceleración a y, por lo tanto, a la del movimiento del sistema. Es el producto de la masa m y la aceleración a. Como se asume que el sistema a ser calculado se mueve en dirección positiva, la fuerza de inercia es precedida por un signo negativo. Aplica lo siguiente: ${{F}_{\tau }}=-m\cdot a$

Fuerza auxiliar de d'Alembert en un cuerpo en movimiento